Flexible Couplings.—Shafts that are out of alignment (
misalignment) laterally or angularly can be connected by any of several designs of
flexible couplings. Such couplings also permit some degree of axial movement in one or both shafts. Some couplings use disks or diaphragms to transmit the torque. Another simple form of flexible coupling consists of two flanges connected by links or endless belts made of leather or other strong, pliable material. Alternatively, the flanges may have projections that engage spacers of molded rubber or other flexible materials that accommodate uneven motion between the shafts. More highly developed flexible couplings use toothed flanges engaged by correspondingly toothed elements, permitting relative movement.
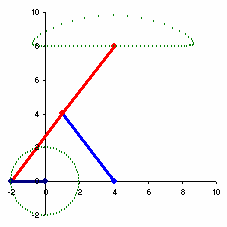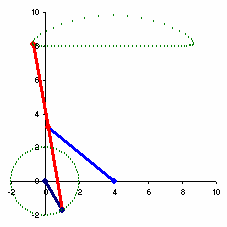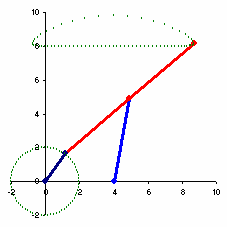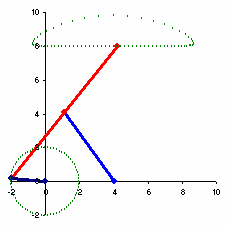
These
flexible couplings require lubrication unless one or more of the elements is made of a self-lubricating material. Other couplings use diaphragms or bellows that can flex to accommodate relative movement between the shafts. The Universal Joint.—This form of coupling, originally known as a
Cardan or Hooke's coupling, is used for connecting two shafts the axes of which are not in line with each other, but which merely intersect at a point. There are many different designs of universal joints or couplings, which are based on the principle embodied in the original design. One wellknown type is shown by the accompanying diagram.
As a rule, a
universal joint does not work well if the angle α (see illustration) is more than 45 degrees, and the angle should preferably be limited to about 20 degrees or 25 degrees, excepting when the speed of rotation is slow and little power is transmitted. Variation in Angular Velocity of Driven Shaft: Owing to the angularity between two shafts connected by a universal joint, there is a variation in the angular velocity of one shaft during a single revolution, and because of this, the use of universal couplings is sometimes prohibited. Thus,
the angular velocity of the driven shaft will not be the same at all points of the revolution as the angular velocity of the driving shaft. In other words, if the driving shaft moves with a uniform motion, then the driven shaft will have a variable motion and, therefore, the universal joint should not be used when absolute uniformity of motion is essential for the driven shaft.
Determining Maximum and Minimum Velocities:
If shaft A (see picture below) runs at a constant speed, shaft B revolves at maximum speed when shaft A occupies the position shown in the illustration, and the minimum speed of shaft B occurs when the fork of the driving shaft A has turned 90 degrees from the position illustrated. The maximum speed of the driven shaft may be obtained by multiplying the speed of the driving shaft by the secant of angle α. The minimum speed of the driven shaft equals the speed of the driver multiplied by cosine α. Thus, if the driver rotates at a constant speed of 100 revolutions per minute and the shaft angle is 25 degrees, the maximum speed of the driven shaft is at a rate equal to 1.1034 ~ 100 = 110.34 R.P.M. The minimum speed rate equals 0.9063 ~ 100 = 90.63; hence, the extreme variation equals 110.34 − 90.63 = 19.71 R.P.M.
Some contents from wikipedia.org
Comments