Cam design
Classes of Cams
may, in general, be divided into two classes: uniform motion cams and accelerated motion cams. The uniform motion cam moves the follower at the same rate of speed from the beginning to the end of the stroke; but as the movement is started from zero to the full speed of the uniform motion and stops in the same abrupt way, there is a distinct shock at the beginning and end of the stroke, if the movement is at all rapid. In machinery working at a high rate of speed, therefore, it is important that cams are so constructed that sudden shocks are avoided when starting the motion or when reversing the direction of motion of the follower.Cams
The uniformly accelerated motion cam is suitable for moderate speeds, but it has the disadvantage of sudden changes in acceleration at the beginning, middle and end of the stroke. A cycloidal motion curve cam produces no abrupt changes in acceleration and is often used in high-speed machinery because it results in low noise, vibration and wear. The cycloidal motion displacement curve is so called because it can be generated from a cycloid which is the locus of a point of a circle rolling on a straight line.
Cam Follower Systems
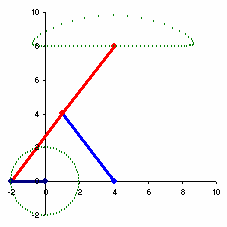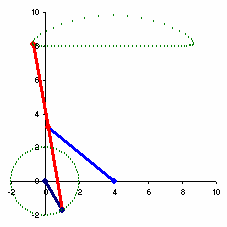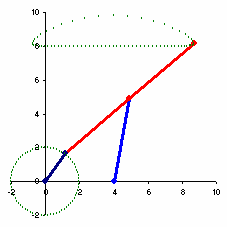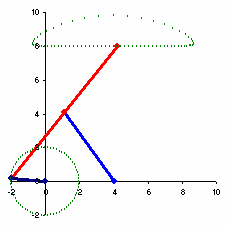
The three most used cam and follower systems are radial and offset translating roller follower, Figs. 1a and 1b; and the swinging roller follower, Fig. 1c. When the cam rotates, it imparts a translating motion to the roller followers in Figs. 1a and 1b and a swinging motion to the roller follower in Fig. 1c. The motionof the follower is, of course, dependent on the shape of the cam; and the following section on displacement diagrams explains how a favorable motion is obtained so that the cam can rotate at high speed without shock.
The arrangements in Figs. 1a, 1b, and 1c show open-track cams. In Figs. 2a and 2b the roller is forced to move in a closed track. Open-track cams build smaller than closed-track cams but, in general, springs are necessary to keep the roller in contact with the cam at all times. Closed-track cams do not require a spring and have the advantage of positive drive throughout the rise and return cycle. The positive drive is sometimes required as in the case where a broken spring would cause serious damage to a machine.
Pressure Angle and Radius of Curvature
The pressure angle at any point on the profile of a cam may be defined as the angle between the direction where the follower wants to go at that point and where the cam wants to push it. It is the angle between the tangent to the path of follower motion and the line perpendicular to the tangent of the cam profile at the point of cam-roller contact.
The size of the pressure angle is important because:
The maximum pressure angle αm should, in general, be kept at or below 30 degrees for translating-type followers and at or below 45 degrees for swinging-type followers.
These values are on the conservative side and in many cases may be increased considerably, but beyond these limits trouble could develop and an analysis is necessary.
Radius of Curvature
The minimum radius of curvature of a cam should be kept as large as possible
Undercutting cannot occur at the concave portion of the cam profile (working surface), but caution should be exerted in not making the radius of curvature equal to the radius of the roller follower. This condition would occur if there is a cusp on the displacement diagram which, of course, should always be avoided. To enable milling or grinding of concave portions of a cam profile, the radius of curvature of concave portions of the cam, Rc = ρmin + rf, must be larger than the radius of the cutter to be used.
Cam Forces, Contact Stresses, and Materials
After a cam and follower configuration has been determined, the forces acting on the cam may be calculated or otherwise determined. Next, the stresses at the cam surface are calculated and suitable materials to withstand the stress are selected. If the calculated maximum stress is too great, it will be necessary to change the cam design.
Such changes may include:
The main factors influencing cam forces are:
Cam Materials: In considering materials for cams it is difficult to select any single material as being the best for every application. Often the choice is based on custom or the machinability of the material rather than its strength. However, the failure of a cam or roller is commonly due to fatigue, so that an important factor to be considered is the limiting wear load, which depends on the surface endurance limits of the materials used and the relative hardnesses of the mating surfaces.
Want to read more details? Check this ... mechanical design handbook store
source: googlebooks
Recommended articles related to Cam Design
may, in general, be divided into two classes: uniform motion cams and accelerated motion cams. The uniform motion cam moves the follower at the same rate of speed from the beginning to the end of the stroke; but as the movement is started from zero to the full speed of the uniform motion and stops in the same abrupt way, there is a distinct shock at the beginning and end of the stroke, if the movement is at all rapid. In machinery working at a high rate of speed, therefore, it is important that cams are so constructed that sudden shocks are avoided when starting the motion or when reversing the direction of motion of the follower.CamsThe uniformly accelerated motion cam is suitable for moderate speeds, but it has the disadvantage of sudden changes in acceleration at the beginning, middle and end of the stroke. A cycloidal motion curve cam produces no abrupt changes in acceleration and is often used in high-speed machinery because it results in low noise, vibration and wear. The cycloidal motion displacement curve is so called because it can be generated from a cycloid which is the locus of a point of a circle rolling on a straight line.
Cam Follower Systems
The three most used cam and follower systems are radial and offset translating roller follower, Figs. 1a and 1b; and the swinging roller follower, Fig. 1c. When the cam rotates, it imparts a translating motion to the roller followers in Figs. 1a and 1b and a swinging motion to the roller follower in Fig. 1c. The motionof the follower is, of course, dependent on the shape of the cam; and the following section on displacement diagrams explains how a favorable motion is obtained so that the cam can rotate at high speed without shock.
The arrangements in Figs. 1a, 1b, and 1c show open-track cams. In Figs. 2a and 2b the roller is forced to move in a closed track. Open-track cams build smaller than closed-track cams but, in general, springs are necessary to keep the roller in contact with the cam at all times. Closed-track cams do not require a spring and have the advantage of positive drive throughout the rise and return cycle. The positive drive is sometimes required as in the case where a broken spring would cause serious damage to a machine.
Pressure Angle and Radius of Curvature
The pressure angle at any point on the profile of a cam may be defined as the angle between the direction where the follower wants to go at that point and where the cam wants to push it. It is the angle between the tangent to the path of follower motion and the line perpendicular to the tangent of the cam profile at the point of cam-roller contact.
The size of the pressure angle is important because:
- Increasing the pressure angle increases the side thrust and this increases the forces exerted on cam and follower.
- Reducing the pressure angle increases the cam size and often this is not desirable because:
- The size of the cam determines, to a certain extent, the size of the machine.
- Larger cams require more precise cutting points in manufacturing and, therefore, an increase in cost.
- Larger cams have higher circumferential speed and small deviations from the theoretical path of the follower cause additional acceleration, the size of which increases with the square of the cam size.
- Larger cams mean more revolving weight and in high-speed machines this leads to increased vibrations in the machine.
- The inertia of a large cam may interfere with quick starting and stopping.
The maximum pressure angle αm should, in general, be kept at or below 30 degrees for translating-type followers and at or below 45 degrees for swinging-type followers.
These values are on the conservative side and in many cases may be increased considerably, but beyond these limits trouble could develop and an analysis is necessary.
Radius of Curvature
The minimum radius of curvature of a cam should be kept as large as possible
- to prevent undercutting of the convex portion of the cam
- to prevent too high surface stresses. Figs. 3(a), (b) and (c) illustrate how undercutting occurs.
Undercutting cannot occur at the concave portion of the cam profile (working surface), but caution should be exerted in not making the radius of curvature equal to the radius of the roller follower. This condition would occur if there is a cusp on the displacement diagram which, of course, should always be avoided. To enable milling or grinding of concave portions of a cam profile, the radius of curvature of concave portions of the cam, RCam Forces, Contact Stresses, and Materials
After a cam and follower configuration has been determined, the forces acting on the cam may be calculated or otherwise determined. Next, the stresses at the cam surface are calculated and suitable materials to withstand the stress are selected. If the calculated maximum stress is too great, it will be necessary to change the cam design.Such changes may include:
- increasing the cam size to decrease pressure angle and increase the radius of curvature
- changing to an offset or swinging follower to reduce the pressure angle
- reducing the cam rotation speed to reduce inertia forces
- increasing the cam rise angle, β, during which the rise,h, occurs
- increasing the thickness of the cam, provided that deflections of the follower are small enough to maintain uniform loading across the width of the cam
- using a more suitable cam curve or modifying the cam curve at critical points
The main factors influencing cam forces are:
- displacement and cam speed (forces due to acceleration)
- dynamic forces due to backlash and flexibility
- linkage dimensions which affect weight and weight distribution
- pressure angle and friction forces
- spring forces
Cam Materials: In considering materials for cams it is difficult to select any single material as being the best for every application. Often the choice is based on custom or the machinability of the material rather than its strength. However, the failure of a cam or roller is commonly due to fatigue, so that an important factor to be considered is the limiting wear load, which depends on the surface endurance limits of the materials used and the relative hardnesses of the mating surfaces.
Want to read more details? Check this ... mechanical design handbook store
source: googlebooks
Recommended articles related to Cam Design
- Timing Diagram (Part 1 - No Overlap Movement)
- Timing Diagram (Part 2 - Maximum acceleration calc...
- Timing Diagram (Part 3 - Cycloid Cam Profile Analy...
- Timing Diagram (Part 4 - Timing Diagrams Compariso...
- Polynomial Cam Function (Introduction) - Part 1
- Polynomial Cam Function (Derivation of Fifth-degre...
- Polynomial Cam Function (Fifth-degree polynomial c...
- Polynomial Cam Function (Fifth-degree polynomial E...
Comments
does it really? then why is it called a uniform acceleration cam at all?
and are you sure the accn also changes in the middle of the stroke?
The uniformly accelerated (constant acceleration) motion cam has a curve known as parabolic curve. It has constant positive and negative accelerations. It has an abrupt change of acceleration at the terminals and the transition point, which makes it undesirable except at low speeds.
See Parabolic cam profile (s, v and a)
We can see from the figure that the jerk is infinity at the beginning, middle and end of the stroke, while cycloidal motion cam curve has finite value of jerk.
See Jerk comparison between Parabolic and Cycloid
Hope this answers your query.
Ake.